
Tomorrow is the fall midterm for my Algebra 2 Honors class. It covers everything from the start of the year: the real number system and its properties, solving equations with rational coefficients, everything about functions and graphical analysis, systems of linear and non-linear inequalities, and 3x3 systems of equations. That's quite a large chunk of material for anyone, let alone students who were below grade level less than 2 years ago.
DCP students are notorious for being able to do well in the short term, but completely falling apart in the long term (>3 weeks or so). Traditionaly, the majority of a class will get a C or an F (no Ds at DCP!) on a midterm or final, even if they had been doing well all along. We haven't figured out yet how to help them do better on large cumulative exams. Sometimes it feels like their brains are a cup that can hold a fixed amount of liquid - as soon as you pour more in, the rest splashes out to make room. For this reason, final exams are only 10% of the class grade in all freshmen classes, and it goes up by 5% a year. We need our seniors to be prepared to take college classes where the final is 25 - 30% (or even more) of the grade, and to understand how significant that is.
I don't know if their retention problems mean that they did not really understand the material in the first place, or if it means that they still don't really understand how to study (or how you need to study differently for this kind of exam), or if there is something else going on. I was helping a student review today, who admittedly has been struggling to do well, but usually has a pretty good sense of what's going on. He got confused on a problem, so I posed a simpler problem (or so I thought...) to try and help him understand. I wrote on the board: if f(x) = x + 2, what is f(3)? He looked at me like he had no idea what I was talking about. Honestly, I am amazed by this. I have no way to explain why he couldn't answer that question. I do have one observation: when students are struggling with one difficult concept, they seem to be unable to access previously learned concepts at the same time, even if they could use that concept well in a different context. Maybe it's like being able to toss and catch different objects individually with no problem, but dropping them all when trying to juggle.
It makes sense to me that students would forget complex formulas or methods over time - this happens to me too. But, if they learned them properly, a little bit of review should bring them right back. However, when students don't recall foundational concepts (I had a calculus student once ask me, while studying for the final, what a derivative was), I don't know how to help them, except to implore them to see me for as much extra help as possible.
So, we'll see tomorrow how it goes. I'm predicting a bloodbath, but I hope they'll defy the trends. If anyone has ideas about how to better prepare students for cumulative tests, please share them in the comments section.
Update:
Here are the scores:
10| 2
9| 7
8| 0 1 5 8 8
7| 1 2 2 2 4 4
6| 2 5 7 8
5| 2 4 5
4| 9
3|
2|
1|
Well, as predicted, the scores were much lower than a typical unit test. At least the majority of the class still passed, and my top student is still getting over 100%! She's an algebra machine.
Monday, November 27, 2006
I'm more afraid of the midterm than they are...
Posted by
Dan Wekselgreene
2
comments
![]()
Labels: algebra 2, cumulative exams, students
Wednesday, November 22, 2006
Next Lesson: 3x3 Systems Word Problems (and Thanksgiving!)
I just finished the class - last period of the last day before Thanksgiving break, right after a special double-length lunch with music, dancing, and staff v. student volleyball and soccer. Given all that, the class went amazingly well. They were wound up at first and were having "focus problems" (my favorite euphamism), but when we got to the competition, they really got down to work. So the lesson..
After reviewing the homework, students did a warm-up of solving word problems with 2x2 systems. They still have trouble with converting from verbal statements to equations for things like "my second number is 3 more than 6 times my first number". To help them through those, I ask them to try a specific numeric example (reducing the level of abstraction by that step seems to help them see the relationship). I say "imagine my number is 10 - what is 3 more than 6 times 10?" They say 63, and then I ask them how they figured that out. That is usually enough to get them to write out the equation.
After this, we worked together on a word problem that uses a 3x3 system as its model. In this example, the last equation was in a different form (where one variable was already isolated), and they saw how substitution would let them create a 2x2 system, instead of just using linear combination as we had been doing. I'm trying to get them to be as flexible as possible in their problem solving; there are different methods, and you should pick the method that applies best to the given problem.
This left about 35 minutes for students to get into groups of 3 and do a word problem relay. Each group got problem 1 to start with; when they solved it, they brought it to the front for me to check. If they were correct, they got problem 2, and so on. There were 4 problems - I told them they'd get 5 bonus homework points if they solved all 4. At this point, most of the students worked really well (except for one chatty group) and most groups got to the third or forth problem, but none solved the forth in time.
For homework, I gave them a review packet for the Midterm, which will be next Tuesday, when they return. You can imagine how excited they were about that! It's five pages long, and I suggested that they do one per day to keep their math brains running.
It was a nice day today overall, and this little break always comes just in time to keep us all (teachers and students) sane and friendly.
Posted by
Dan Wekselgreene
0
comments
![]()
Labels: algebra 2, lesson, systems of equations
Sunday, November 19, 2006
Our Gorgeous Department

Click here to see the rest of the staff. Signed 8x10 glossies are available by request!
Posted by
Dan Wekselgreene
0
comments
![]()
Labels: photos
Next Lesson: 3D Graphing (better than the PS3!)
In this lesson, I will take the class to our computer lab so we can work with the OS X Grapher application. This is the program I've been using to generate all the 2D graphs I give them on worksheets. It can do 3D graphs as well, with nice lighting and rotation. You can even graph implicit functions and relations - I like plugging in random equations to see what kinds of shapes it can make.
We'll start by looking at the 3 axes and see what the planes x, y, and z = 0 look like, as well plotting an ordered triple.
Then, I'll show them what the graph of ax + by + cz = d looks like, and how three planes can intersect at a single point (this being the solution to a 3x3 system of equations).
We'll also look at systems with no solution (i.e. at least two of the planes are parallel) or an infinite number of solutions (all three intersect at the same line).
I think that seeing this will help them visualize 3D graphing much better than me trying to draw it on the board.
During the demonstration, I will be teaching them how to enter functions in to Grapher. After the demonstration, I will put two 3x3 systems on the board. One has a solution and the other doesn't. They must first decide which does by graphing both and determining which has a point of intersection. Once they decide, they must solve the system algebraically, and then plot the solution to see if it falls on the point of intersection of the planes.
After this, we'll go medium-tech (i.e. just using their TIs). I will show them how to use the "stat plot" feature, and we will plot the points (-1, -10), (1, -18), and (5, 14). I will ask them if they can figure out the function of the parabola that passes through those three points. I don't expect them to be able to figure this out on their own, so I will lead them through plugging in the points into the standard form of a quadratic function, which will generate a 3x3 system of equations. We'll solve this together to find that a = 2, b = -4, and c = -16. Graphing the parabola y = 2x^2 - 4x - 16 will confirm the work.
Hopefully there will be time for them to try this on their own as practice. I'll ask them to find the function of the parabola that passes through (-2, 0), (-1, -6), and (3, -10). This is definitely a lot for one lesson, and I've never done this before (our lab is new!), so I'll have to see how it goes.
Posted by
Dan Wekselgreene
3
comments
![]()
Labels: algebra 2, Grapher, lesson, systems of equations
Wednesday, November 15, 2006
Real World Applications
What math teacher hasn't heard "when will we use this in real life?" a thousand times already? Typically you hear this when the going gets tough, but sometimes it's asked as a genuine question - not just teacher-baiting. I've posted on this before, and there was quite a bit of response. It's still an open question, obviously, and based on some discussion we had at our staff meeting today, I wanted to approach the question from another angle.
Though students will always be using their numeracy skills, I don't try to convince them that they will be using algebra, geometry, or trig in their "daily lives", because it is simply not true. I do write word problems that use their names and put them in familiar situations, but just because it is fun for them and me - not because I think this will dramatically increase their buy-in and engagement, or their understanding of mathematical concepts. I talk about how math helps you build logical thinking and problem solving skills, but this doesn't help much for the unmotivated students.
The idea we generated today was not to focus on how students might use advanced math concepts in their own daily lives, but to teach them how they are used by professionals in different lines of work. My quadratic functions unit is coming up. Sure, basketballs fly through the air in lovely parabolas, but does that really create a meaningful connection for a kid, even if they love sports? Maybe, but I doubt it. Does a basketball player quickly use x=-b/2a before taking a shot? Instead, what about some applications of quadratic functions that show how they are really used by scientists, engineers, sociologists, astrophysicists, biologists, etc. Maybe a 5 minute, detailed presentation on how headlights and satellite dishes work? It may not connect to students on a personal level, but it could help them see the value math has to society, and why it's worth studying.
So that's my new task: gathering relevant, interesting applications of math concepts that our department can use for each unit in algebra, geometry, trig, and calculus. I have some ideas already, but I would love to hear other people's thoughts on this. Do you think this will be beneficial? Do you know of good resources regarding these questions? Do you have good examples of applications for specific concepts?
Posted by
Dan Wekselgreene
8
comments
![]()
Labels: real world, students
Tuesday, November 14, 2006
Next Lesson: 3x3 Systems of Equations
Up next are those pesky systems of three equations with three variables. We are going to work on solving them by linear combination. I've decided not to cover matrices in this class because of the time it would take to teach it to a level that had any meaning to the students. I also don't think that they have much application up through Calculus (at least, not enough to justify taking time away from some of the other critical topics).
The class will start with a review of 2x2 systems, including systems with no solution or an infinite number of solutions. They will solve these algebraically and graphically; I want to see if they can draw their own conclusions first about what algebraic results like "0 = 3" or "0 = 0" imply graphically.
After this, I'll do direct instruction on these ideas, followed by a demonstration of how to solve a 3x3 system by linear combination. The steps I'll give them:
- Decide which variable is easiest to eliminate.
- Eliminate that variable from one pair of equations.
- Eliminate the same variable from a different pair of equations.
- Write the resulting equations as a 2x2 system and solve it.
- If the 2x2 has 0 or infinitely many solutions, the same is true about the 3x3 and you can stop there.
- See if you can use division to simplify the new equations.
- Plug in the solutions to one of the original equations and solve for the third variable.
- Check by plugging in the solution to the other two original equations.
After that, it's just a matter of practice grinding through a few systems. Homework will be more of the same, with a +10 point mega bonus extra credit problem (solving a 4x4 system).
In the following class, we will use the Grapher application in our Mac lab to see the planes that are produced by an equation with x, y, and z, and to understand what an ordered triple means. We will also be able to see how planes can intersect and so forth, and maybe play around with some more 3-D graphing. Grapher is a great application - check it out if you can.
Posted by
Dan Wekselgreene
0
comments
![]()
Labels: algebra 2, lesson, systems of equations
Monday, November 13, 2006
Next Lesson: Systems of Inequalities
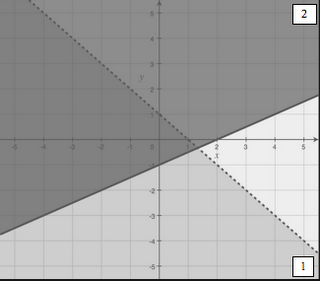
In this lesson, we will go beyond graphing linear inequalities to graphing systems of inequalities, including non-linear inequalities (we'll use absolute value and quadratic equations, as those as the non-linear functions we practiced in the last unit).
Just like in the last lesson, we'll start with a visual activity. Students are given a graph of a system of inequalities, with the two inequalities labeled "1" and "2". They are given a bunch of points, and must determine if the point satisfies the first, the second, both, or neither, and then plot out a "1", "2", "B", or "N" at the coordinates. I want them to not just know that the overlapping region is the solution, but to be able to identify which inequality in the system is not satisfied by a given non-solution point.
At this point, there will be some direct instruction on how to graph a system of inequalities, and reinforcement that the overlapping region is the set of all points that satisfies all inequalities in the system. I will also show them how to graph non-linear inequalities by graphing the "border function" (paying attention to whether it is a solid or dashed line), and then plugging in a test point to determine which region to shade.
After this, students will work independently to practice. I think these will be a bit tough for them because each problem has a little wrinkle in it. I didn't want to give them a bunch of simple lines - that wouldn't be any fun! We'll see how they do. I hope they get it, because I want to move on to the next topic in the following lesson and not spend much time reviewing.
The lesson will be posted at ILoveMath.org.
Posted by
Dan Wekselgreene
0
comments
![]()
Labels: algebra 2, lesson, system of inequalities
Friday, November 10, 2006
Next Lesson: Graphing Linear Inequalities

We are finally moving on from the functions unit into a brief unit on systems of equations and inequalities. Last year, I included linear programming in this unit, because it is a great application not just of systems of inequalities, but being able to think critically about a problem, convert verbal information into a mathematical model, and so forth. Unfortunately, I think this topic will be a casualty of going so deep with functions this year. Every time I don't teach some topic, I feel like I am denying them access to ideas that other students will know. Maybe I can squeeze in a one-lesson overview just so they see the idea.
After the unit test, I gave students a homework to review graphing linear functions (horizontal and vertical, slope-intercept, standard form, finding equations of a line, etc.). I think they were happy to get something that was so "easy" for a change.
In this lesson (I actually taught it yesterday), students first were given a Do Now to explore the idea of shading a solution region. I asked them to graph x - 2y = 8. Then, I gave them a bunch of points and the inequality x - 2y < 8, and asked them to algebraically determine if each point was a solution to the inequality. If it was, they had to plot a Y at the coordinates. If not, they had to plot an N. Seeing all the Ys on one side of the line (and on the line itself) and the Ns on the other helped get across the idea of the solution region. Last year, they were taught to shade the solution region in, but I wanted to make sure that they understood what the shading really means. We talked about how the picture would look different if the inequality symbol flipped, or was exclusively greater or less than.
After we established this concept, I just did some direct instruction and showed them how to graph linear inequalities in various forms: x < c; y < c; |x| < c; |y| < c; y < mx + b; ax + by < c. After each example I did, they did a similar example on their own (switching the type of inequality symbol).
The class ended with some individual/pair practice of graphing. The practice also included determining visually if a point is a solution (given the graph), and then confirming this algebraically.
The homework includes more of the same type of practice. It also has a couple of non-linear inequality challenge problems (like y < (x - 3)^2 + 4) to help them work on their synthesis skills.
I'll post the whole lesson on ILoveMath.org
Posted by
Dan Wekselgreene
0
comments
![]()
Labels: algebra 2, lesson, linear inequalities
Tuesday, November 07, 2006
Functional Success

Break out the champagne! Here are the results to the Functions and Relations unit test:
10| 3
9| 0 1 2 3 3 7 7 8 8
8| 0 0 4 8
7| 0 1 7 8 9 9
6| 8
5|
4| 8 8
3|
2|
1|
I'm really happy that they did so well. It's good to have some real successes - both for me and for the students. Of course, now I worry that the test was too easy! I'm posting it at ILoveMath if anyone wants to look at it or use parts of it (or you can email me for a copy). I would love to hear any comments on it, because I redid the whole unit this year, and I strayed quite a bit from the state standards.
Posted by
Dan Wekselgreene
0
comments
![]()
Monday, November 06, 2006
I'm really not obsessed with transformers, I promise!
...but this is just too funny. Click in the box to play the video.
Posted by
Dan Wekselgreene
0
comments
![]()
Labels: transformers
November Surprise
Something happened this weekend that I don't think has ever happened in any class in the past 6 years: every student in my 3rd period Algebra 2 regular class passed the unit test. This has happened occasionally on smaller quizzes, but never on a unit test. If you have read enough of my posts to understand what DCP students are like, you'll realize that this is something of a minor miracle. This test was on systems of equations and inequalities, including a word problem and a 3x3 system of equations (with either no solution or an infinite number of solutions). The test wasn't killer, but it definitely wasn't a cakewalk.
I don't usually post about my Algebra 2 regular classes because I don't plan those lessons (we share preps to reduce workload and to ensure that all students learn the same concepts and are assessed the same way). To be honest, because I don't plan the curriculum, I don't spend as much time as I should reflecting on the progress of those classes. But I have some really great kids in those classes too (I got really lucky this year - I have almost no, shall we say, "negative personalities").
Anyway, I told my class the good news today, and I was dead right about the first words that would erupt from the crowd. Say it with me: "Do we get a pizza party?"
Posted by
Dan Wekselgreene
0
comments
![]()
Sunday, November 05, 2006
The Phantom of the Quinceanera

This weekend, I went to my first quinceanera party. The theme, though I'm not sure why exactly, was Phantom. The birthday girl was a student I had in Numeracy last year, who is a great kid, but has extreme math phobia/anxiety/learning disabilities. She grew comfortable with me as a teacher, and so we were able to have a productive year. Though it is hard to watch a student who works so hard, and is so good natured about everything, struggle so mightily with such basic concepts.
In any case, I was there with about four or five other teachers at the "Reserved for DCP Teachers" table, which was placed up in front, right among the immediate family tables. Both the girl and her mother were very happy that we had come, and it was a great feeling. She was wearing an unbelieveable dress - imagine a relatively small, thin girl stuck in the middle of a 5-foot diameter gold satin gown with ruffles. She told us it made her hips hurt! The 7 damas were wearing gold satin gowns and masks as well, and the 7 male partners were wearing tuxes with gold shirts of some sort, masks, and black capes. There must have been at least 50 other students there - more kept showing up as the night progressed. The party started with food and mariachis playing for a couple of hours. Then, the 15 kids did a couple of choreographed dances to some kind of techno-phantom remix. They left for a costume change and came back to do another dance, and then did another change back into their original dress. Apparently, these dances are choreographed specially for each quinceanera. I've always had students in the past miss class or not get homework done becase they had "quinceanera practice". Now I understand a lot better what kind of work actually goes into making this happen.
Overall, it was a lot of fun. All the students came up to us and shook hands, gave hugs, and were genuinely happy to see us and talk with us. A couple of my Algebra 2 honors students saw me and told me they were getting together later this weekend at the library to study for the test! I even got to see a bunch of former students who have either graduated, or ended up not staying at DCP. This kind of welcoming, community spirit is not only great to be part of - it's really interesting to me... thinking back to my Bar Mitzvah (which is really a very similar event, when it comes down to it), my friends and I would not have been quite so happy to see our teachers there. It would have felt weird, and I definitely would not have run up to my math teacher and given her a hug. No matter how rough our classes are at times, or what type of discipline problems we deal with at school, I'm always amazed by how connected our students and their families are to us, to the school, and to each other.
Update:
At school today, students kept coming up to us and asking why we left the party so early. They wanted to dance with us!
Posted by
Dan Wekselgreene
1 comments
![]()
Labels: students
Friday, November 03, 2006
Next Lesson: Quiz 3 and Study Groups
The previous lesson on solving equations and inequalities graphically was very difficult for the students, and I ended up having to do an entire extra lesson of just practice. They worked very hard and I heard quite a few "my head hurts!" comments. Even with that effort, they were taking much longer than I had predicted to do the work, so I ended up pushing the quiz off until the next lesson (this afternoon).
It's interesting: my students are easily able to solve equations such as 2|3x - 4| + 5 = 9 this way, by identifying points of intersection of the functions f(x) = 2|3x - 4| + 5 and g(x) = 9, and looking at their x-values.
As soon as the problem changes to an inequality like 2|3x - 4| + 5 > 9 , they get lost. There seems to be a disconnect between knowing where the f(x) values are greater than the g(x) values (which they understand), and being able to identify the set of x-values that produce those y-values. Many of the students seem to flip back and forth between the x- and y-values and get confused with what they are doing.
In the review lesson, I had them start again with writing the solution in inequality and interval notations when given a number line. This seems to have helped some of them make the connection. I think that many more students were getting the idea by the end of class. I'll see how much they have actually learned when I grade the quizzes today.
After the quiz, they will be working in study groups, which is one of my goals for this year. Part of their homework for today was to look at the different sections that will be on the test (which I listed for them), to flip through notes and old quizzes to remember what types of problems are in each section, and then to rank those sections based on how well they think they are prepared. I resisted creating a review packet for them for when they will be working in their study groups today. Instead, they must compare their rankings and decide as a group what topics to study. Then, they need to find example problems in their notes, homework, and old quizzes. This is risky, because students (even my lovely honors kids) without a concrete assignment (like a worksheet) tend to lose focus and not use their time well. But that is what I want to coach them on, so we'll see. I did make a short review sheet with some key problems for them to complete as homework. I hope to take away more of this scaffolding by the end of the year.
Here are the sections on the Unit 2 Test:
1) Functions and relations
2) Function notation & function composition
3) Representational fluency (using equations, tables, arrow maps, and graphs)
4) Operations on functions (adding, subtracting, multiplying)
5) Domain and range
6) Piecewise functions
7) Translating functions
8) Transforming functions
9) Solving equations and inequalities graphically
Update:
Quiz 3 scores:
10| 0 0
9| 2 6 8 8 8
8| 0 2 6
7| 6 6 8
6| 0 4 4 6 8 8
5| 0 4
4|
3| 2
2|
1|
The class seems to have split into those that really understood and those who didn't. There's something about inequality signs that just drives some kids bonkers.
Posted by
Dan Wekselgreene
0
comments
![]()
Labels: algebra 2, lesson, study groups
Wednesday, November 01, 2006
Critical Thinking

I have this Escher drawing on the desktop of my laptop. When showing grades to students, one noticed the picture and said, "Is that your house?".
Posted by
Dan Wekselgreene
1 comments
![]()
Labels: students
